My last post was in response to much talk of positive fedbacks and instability. I showed an active circuit (borrowed from Bernie Hutchins) which wass table and would emulate the usual equation of feedback in the conetxt of climate sensitivity:
ΔTeq = ΔF/( 1/λ0 – Σci)
My main observation is that this is just like Ohm's Law, with the feedback coefficients c as conductances in parallel. The need to resort to active feedback comes from the fact that some c's, corresponding to positive feedback, may be negative. But the basic simplifying idea off adding conductances still applies.
In a much-cited review paper, Roe 2009, roe argues that feedbacks should be seen as Taylor series in disguise. I think he could have said just chain rule, since he uses only first order. But I want to develop this, because it is the basis for the method used in an also much-cited (including AR4) paper by Soden and Held, 2006. Lord M's latest post is based on the claim that S&H have made an error, but he really has no idea of what they did. So I'll try to say something about that here.
We're thinking about equilibrium climate sensitivity
S = ΔTS/ ΔF
ΔTS is a sustained change in global average surface temperature caused by change of forcing ΔF. But if the change in T changes other quantities xi with i over some range, and these x generate new changes in F, e may need to take this into account. The chain rule expression says
dF = ∂F/∂xi ∂xi/∂TS dTS
Note that although we are looking for dependence of T on F, we expand the denominator F in the ratio.
The quantities x are those that contribute to F through some radiative relation that is either known or can be worked out by some atmospheric model. There is no great delay here so the calculation is quick. But x are also observable over time, probably in a GCM, so the second factors can be got by observation over time. This was the basis of the S&H method. They looked at a number of GCM implementations of the SRES A1B scenario over a century. In this, GHGs rose for a period of some decades, but then rose no further, giving a reasonable version of equilibrium change.
The variables S&H looked at were temperature T, water vapor, and surface albedo. They list various reasons why the approach is not suitable for cloud feedback, and so deduced that as the remainder, since the feedbacks add to inverse sensitivity. This emphasises that the method is for breaking down; you can't get ECS from the components.
The interesting one here is T. It expresses the Planck feedback idea of the previous post, that higher T radiates more energy, lowering (or balancing) F. But what T to use? Lord M could not be shaken in his belief that S&H used the temperature of the "emission layer", about 255K, even though there is not the slightest mention of that in the paper. In fact, it isn't good to use any global average T, since IR emission responds to the local and immediate T, and in a non-linear way.
So S&H broke T into a whole lot of local variables, based on the GDDL GCM grid, depending on time of day and year, latitude and altitude. Here is their description:
To compute Kx, we first calculate the control top-of-the-atmosphere (TOA) radiative fluxes using 3-hourly values of temperature, water vapor, cloud properties, and surface albedo from a control simulation of the GFDL GCM. For each level k, the temperature is increased by 1 K and the resulting change in TOA fluxes determines (∂R/∂T_k).It doesn't say how long, but I presume for a year. That gives the necessary ∂F/∂xi. Then they get the rate of change thus:
The model response for each variable x is computed by differencing the projected climate of years (2000–10) from that of (2100–10); (dx/dTs) = (x(2100-2110) - x(2000-2010))/(Ts(2100-2110) - Ts(2000-2010)). The response is then scaled by the appropriate radiative adjoint (KX = (∂R/∂x)) to yield the climate feedback parameter for that variable, λX = KX(dx/dTs),
The products are formed for each cell/time and then average for total ∂F/∂T ∂T/∂TS to get λT. They then break this down further into what they call Planck feedback λ0, which expresses the effect of chaging T uniformly, and a lapse rate feedback λ0, which expresses the effect of differential T change:
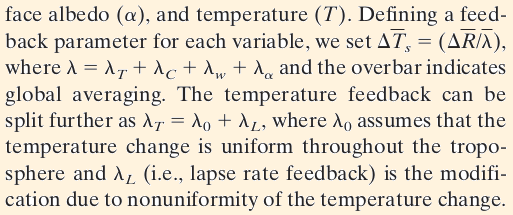
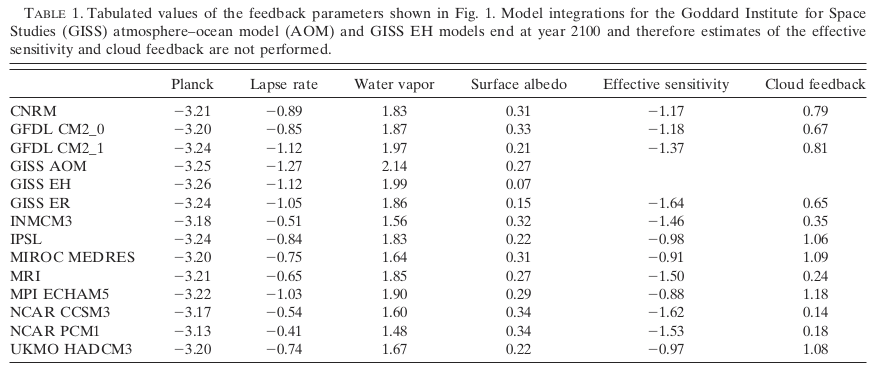
For completeness, here is the S&H table of the feedbacks:
Update. MarkR asked if I could post part of Fig 2. I think it is all quite interesting. Here it is, in two parts:
 |
 |












Could you put up the temperature part of Figure 2 from Soden & Held? It shows how the surface always contributes a bit and how the biggest response in the moist tropics comes from high up, but low down in the dry descending branches of the Hadley cell. There's a world of wonderful detail in there that Monckton is completely and blissfully clueless about.
ReplyDeleteThere's now a similar approach for cloud radiative effects too. Here's how they were calculated in a bunch of models:
http://journals.ametsoc.org/doi/abs/10.1175/JCLI-D-11-00248.1
And recent work using the A-train satellites to observe them:
http://journals.ametsoc.org/doi/abs/10.1175/JCLI-D-15-0257.1
Thanks, Mark,
DeleteI think the whole fig is interesting.
The chain rule rules! Knowing how to apply the chain rule is important for separating variables and being able to reduce a complex set of equations into something simple. http://contextearth.com/2016/07/04/alternate-simplification-of-qbo-from-laplaces-tidal-equations/
ReplyDeleteThanks for posting the figure Nick.
ReplyDeleteMonckton seems to be insisting that the feedback should be determined from the flux change when the temperature at the "peak emission altitude" changes by 1 K. He seems to believe this is around 5 km, although Figure 2 shows that this altitude depends on where you are. If we followed Monckton's idea of just changing the temperature at one level, arbitrarily define a layer to be 100 hPa thick and then see the change in flux, then you get a response of somewhere 0.3-0.33 W m-2 K-1 by following Monckton's idea.
The real value is ~3.1 W m-2 K-1, Monckton thinks it's bigger than the real value, but following his suggestion gives a much smaller number. This is because Monckton doesn't understand the relevance of Planck's Law and that radiative emission or absorption in a gas depends on wavelength, amount of gas etc.
FWIW, a kind of reality check:
DeleteAccording to the trend of RSS TTT v4, the temperature has increased by 0.67 C from 1979 til now.
Applying Stefan-Boltzmann law on this (assuming a effective temperature level of 260 K), suggests that the radiative emission has increased by 2.45 W/m2.
According to the AGGI greenhouse index, the forcing has increased by 1.30 W/m2 during this period. This suggests that there is a total feedback of about 1.15 W/m2 at work..
Olof,
DeleteI think forcing+feedback doesn't necessarily add to OLR. There is also a flux into the ocean.
Of course, I forgot the heat accumulation on Earth. I have estimated that earlier, averaged over the 2005-2015, to be about 0.95 W/m2. Adding that to 1.15 means that the total feedback could be about 2.1 W/m2
DeleteOr have I got something terribly wrong...?
The global heat accumulation estimate increase when looking at more recent periods (2010-2015 = 1.2 W/m2, 2012-2015 = 1.6 W/m2. It looks like we are closing in on a factor 3 between forcing and forcing+feedback...
Thanks a lot to Nick, MarkR and Olof for all these helpful explanations.
ReplyDeleteNick, Could you define what they mean by Effective Sensitivity in Table 1 or simply give the units?
ReplyDeleteThanks in advance.
W m-2 K-1, equation is in the first full paragraph on p3357 here:
Deletehttps://www.gfdl.noaa.gov/bibliography/related_files/bjs0601.pdf