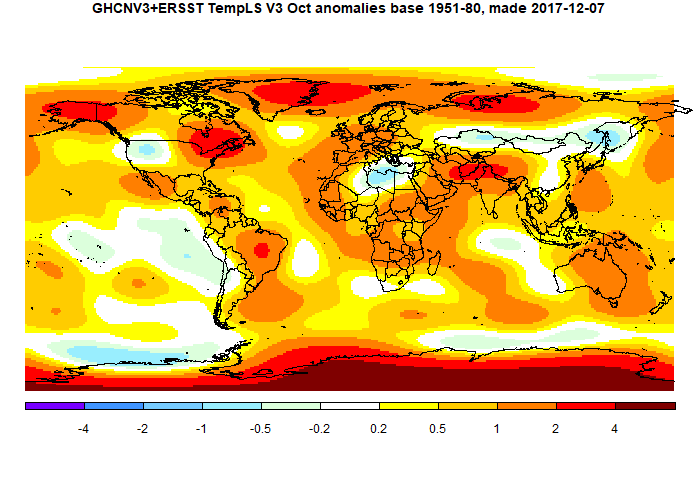
Despite the fall, most of the world looked pretty warm. Warmth in Canada, S America, near East, China. A cool spot in Siberia, but warmth in Antarctica, which contrasts with the earlier NCEP/NCAR report, which showed predominant cold. Here is the temperature map: :
This post is part of a series that has now run for six years. The TempLS mesh data is reported here, and the recent history of monthly readings is here. Unadjusted GHCN is normally used, but if you click the TempLS button there, it will show data with adjusted, and also with different integration methods. There is an interactive graph using 1981-2010 base period here which you can use to show different periods, or compare with other indices. There is a general guide to TempLS here.
The reporting cycle starts with a report of the daily reanalysis index on about the 4th of the month. The next post is this, the TempLS report, usually about the 8th. Then when the GISS result comes out, usually about the 15th, I discuss it and compare with TempLS. The TempLS graph uses a spherical harmonics to the TempLS mesh residuals; the residuals are displayed more directly using a triangular grid in a better resolved WebGL plot here.
The reporting cycle starts with a report of the daily reanalysis index on about the 4th of the month. The next post is this, the TempLS report, usually about the 8th. Then when the GISS result comes out, usually about the 15th, I discuss it and compare with TempLS. The TempLS graph uses a spherical harmonics to the TempLS mesh residuals; the residuals are displayed more directly using a triangular grid in a better resolved WebGL plot here.












Sure enough, climate scientists are starting to use math constructs from solid-state and condensed-matter physics
ReplyDeleteWhat Earth's climate system and topological insulators have in common
Topological insulators are a class of experimental structures that are typically used to investigate reduced dimension quantum effects. Often the carrier transport is confined to interfaces.
The equator poses an interesting analog in that the Coriolis effect reverses sign on either side, thus providing a similar type of interface as occurs with the edge effects of the Quantum Hall Effect.
Both ENSO or QBO follow this kind of behavior in a no-quantum realm.
YouTube simulation
The Antarctic sea ice extent is near a record low for the time of year, as is the global sea ice extent. Could the effect on albedo explain high global temperatures, and the warm pool in Antarctica?
ReplyDeleteYou may have explained this, but I can't remember. Is your new optimized grid going to be your main integration method, or is it replacing the old grid method? Or will it be included along side the others?
ReplyDeleteHi Nick,
ReplyDeletesorry to jump in with an OT here, but I was very interested by your recent on comments about combining distributions using convolution. I think this could address something that I've been trying to find out for a while:
how can you combine the effects of two distributions on the quotient? If I have two , say gaussian distributed, data that are divided : eg x/y , how can I find the distribution of the quotient, or even the product for that matter?
Thanks for any insights you can provide.
Regards, Greg Goodman.
Greg,
DeleteYou can think about it via the logarithm. If the log has a sensible distribution, then the sum/product will combine those through convolution; then you have to hope that antilog will work as well. The condition for that (sensible) is that the domain is bounded well away from zero. But if that is true, then you can work thus:
A/B=Am/Bm+(A-Am)/Bm - Am*(B-Bm)/Bm/Bm+...
that is, just expanding about the means Am, Bm. The ... hides terms that are themselves products of the centered distributions, like (A-Am)*(B-Bm)/Am/Bm, so you would hope first that they are small, or second that they can also be approximated. But the terms shown are tractable, so that is a starting point.
Domain "bounded" away from zero for B means that Bm is large relative to the sd of B, with more conditions about the distribution not having fat tails.