Hadley Cells and Carnot Heat Engine

I've been commenting on a thread at ATTP called "Atmospheric Heat Engines". It's about a paper by Laliberte et al which appeared recently in Science. There is an article about it in the Smithsonian.

The eye-catching thesis is that in a warming climate, the heat engine that drives the poleward circulation, typified by the Hadley Cell, will work with decreased efficiency, because water vapor will not work as well as a working fluid. Their abstract says:

Incoming and outgoing solar radiation couple with heat exchange at Earth’s surface to drive weather patterns that redistribute heat and moisture around the globe, creating an atmospheric heat engine. Here, we investigate the engine’s work output using thermodynamic diagrams computed from reanalyzed observations and from a climate model simulation with anthropogenic forcing.We show that the work output is always less than that of an equivalent Carnot cycle and that it is constrained by the power necessary to maintain the hydrological cycle. In the climate simulation, the hydrological cycle increases more rapidly than the equivalent Carnot cycle. We conclude that the intensification of the hydrological cycle in warmer climates might limit the heat engine’s ability to generate work.

I think there are some global constraints here, as I said in a comment at ATTP. It's something I've written about here over the years, here, here, and here. Overall, I'm uncertain. I've read the paper, and will continue to try to understand it. But meanwhile, I will give my understanding of Carnot cycles, how Hadley cells emulate one, and what role water vapor plays.

The Carnot Cycle

Wiki shows this schematic. You could think of a piston like a coffee plunger, with two plates - source hot, sink cold. With the air initially hot, you place it on the hot plate which is at the same temp. It expands and does work, which staying at the constant temperature of the source. After a while, you take it off the plate. It continues expanding and doing work but now cooling. When it cools to the temp of the sink, you put it on that plate, and now start compressing. During this stage, the entropy that was admitted at the source stage is emitted. When it has gone you withdraw, but keep compressing adiabatically until it warms to the temperature of the source. Repeat...

More formal is this P-V (pressure-volume) diagram from Wiki. The description is here. I'd like to pick up some points. We need the concepts of: Internal energy U. For an ideal gas thus is just cv N T, where N is mass according to the units of cv Enthalpy H = U + PV You can see how the engine does work from the P-V graph. Work is PdV, and while the volume change is the same in expansion and compression, expansion happens at higher pressure. Note that a reserve of mechanical energy is needed. You have to do work to get work.

The heat gained from the source adds directly to the enthalpy. In the first step (1->2), U is constant, so the enthalpy change goes entirely into PV work. In the second, enthalpy doesn't change (adiabatic), so the work done just comes from the change in U. That is the same change in the fourth adiabatic compression stage, so they do no net work. The net work is done by the excess of the first stage over the third.

Hadley Cell

The Hadley cell is an eddy where warm air rises in the tropics (ITCZ), travels at high altitude supplying heat to support TOA IR emission, then descends in mid-latitudes (subtropical ridge) and returns via the surface trade winds. There are Coriolis effects, but the main heat engine effects can be considered without them. The subtropical ridge is a region of high pressure, so the mass of air there is high. Where the cell rises, (ITCZ), the surface pressure is lower, so the mass of air is less. However, it is warmer, so the center of mass is actually higher. To think of it as a Carnot cycle, the first thing needed is the reservoir of mechanical energy. Kinetic energy plays some role, but the main one is hydrostatics. The bouyant rise in the tropics carries the air to a high altitude, whence it travels gradually downhill to the descent phase. The potential energy gained carries it through the cooling phase. It is bouyancy that keeps the low pressure at the equaqtor, and negative bouyancy that pushes air into the pressure maximum at the subtropical ridge. The resulting pressure gradient drives the trade wind stage. So next to the Carnot analogy, thinking first of a dry circulation. I've identified four stages. The heat source is the surface, so the step 1, where the working fluid picks up heat, is far from isothermal. The fluid gains internal energy, while still expanding. The expansion contributes by facilitating the gravitational gradient at high altitude. The (adiabatic, approx) rise stage is enhanced by the internal energy thus gained. The expansion does work to maintain a slightly reduced hydrostatic pressure gradient, in turn maintaining the surface low pressure and the pressure gradient that drives the trades. In the third, high altitude stage, heat is lost to the sink (space) by radiation. In the Carnot cycle, mechanical compression maintained the temperature. Here the gradual loss of altitude has the same effect. Potential energy is the reservoir. In the final stage, the cold air compresses and warms. But it was dense air with relatively less far to descend, so the negative bouyancy allows to to recharge the high pressure zone at the subtropical ridge, keeping up the other end of the surface pressure gradient that drives the trade winds.

Diagrams from Wikipedia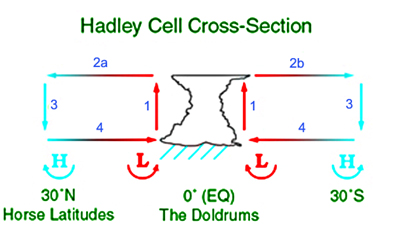

Effect of water vapor

That was a dry Hadley Cell. It's generally reckoned that evaporation and latent heat (LH) are powerful extra drivers. This is partly because wv is bouyant, but also because LH increases the heat carrying capacity of the air.

LH, if you count it as internal energy, is transferred to the air in addition to sensible heat at the trade wind stage, more especially at the later, warmer stage. But the necessary evaporation takes heat from something. Partly sea water, partly air. The cooled air may be further heated by the air-sea temp differential thus created, but still, there is probably less sensible heat taken up than in the dry case.

As I understand it, Lalibertie et al are saying that in fact you can't count the latent heat until it does actually condense. And as global warming proceeds, LH is a greater proportion of internal energy, and this is a limitation.

But obviously water vapor does condense somewhere along the cycle. And most of it will have done so before the end of the bouyant rise. In doing so, it warms (or slows cooling) of the rising air, and allows it to rise to a greater height than it otherwise would have. Or put another way, it helps maintain the surface low pressure, and thus the surface pressure gradient that drives the trade winds. So the fact that the condensation occurs later does not diminish the work available to the cycle.

Conclusions

Well, none. This is a blog, and these are tentative thoughts. What do you all think? Me, I'll read the paper again.