Gore never showed any interest - he just said that he doesn't bet. So it was empty noise. However, for some strange reason, there is now a volley of fantasy articles, attributing a bet to Gore (in which he had no say) and declaring him the loser. The terms of the bet are exceedingly arcane. Since they have to make up some warming forecast, they picked an "IPCC" forecast of 0.3°C warming for the decade.
The first thing to say is that the IPCC made no such forecast. They did, in the AR4 SPM that came out at abaout that time, say this:
For the next two decades, a warming of about 0.2°C per decade is projected for a range of SRES emission scenarios.And it was for surface temperature, not the troposphere measure that Armstrong/Green decided on.
But that itself turns out oddly. They nominate UAH as a measure, which actually has a slightly higher trend over the period than surface measures. Here is the plot
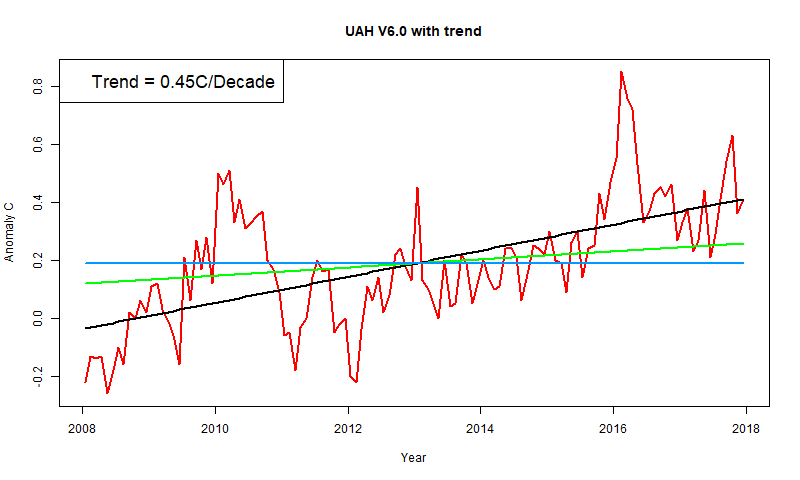
And they are betting with zero trend (blue). The actual was more than double the "IPCC trend". They lose by a mile. But in the WUWT article, at least, they say the OLS trend for the period is 1.53°C/Century. But they prefer a measure they make up called Least Absolute Deviation (LAD).
Well, they would, wouldn't they, because they say that comes out to 1.14°C/Century, and on that basis they declare themselves the winner (since they have pinned Gore with the "IPCC" 3 C/century. Of course it is 2, not 3, so even on that basis they lose. But since they seem to have miscalculated the OLS trend by a factor of 3, I have no faith in their LAD calculation.
On the plot, I've marked a line with slope 1.14°C/century in green, and the Armstrong/Green zero forecast in blue. They all, as fitted lines should, pass through the mean of x and y. See if you think the "LAD" line (1.14) is a better fit. Or whether the no trend forecast is the winner.
Scott Armstrong is a professor of marketing. Kesten Green is a lecturer in Commerce. Neither seems to know much about what the IPCC actually says. And they seem pretty weak in statistics.












Rise of the Innumerates, now playing worldwide.
ReplyDeleteLying comes so easily to these types. That's why they are all aligning behind Trump as someone that they can emulate.
ReplyDeleteIt is just a bald-faced lie - it is incredible, isn't it?
ReplyDeleteIt looks like they made up their pigeon-argument just to get headlines in the fake news media.
I noticed how wrong they got it despite making last minute changes to the "bet" - including a major one nearly nine years after it was originally made.
ReplyDeleteWhat they appear to be doing is pinning the value at the beginning of 2008 to the 2007 annual value. 2008 was well below the trend. This means they are simply finding the best fit to the slope while starting the IPCC line well above the 2008 annual value.
ReplyDeleteThey are also using UAH 6.0 which did not exist in 2008. Ah well.
Eli,
DeleteYes, there is a technical note at the bottom that is hard to follow, but seems to involve some of that. However, their statement in the text
" The OLS trend for the 120 months of the bet was 1.53°C per century"
is clear, unambiguous and wrong. It's bad luck that they chose a decade that starts with a much colder year, and understandable that they want to use information from outside he range to alleviate that. But pinning a trend to a fixed endpoint is the worst way to do that.
The naive way to say whether it has warmed is to take a measure from the end, say a year average, and subtract a measure from the start. OLS is generally preferred because it uses more data and is more stable. You might get a very different result if you choose say the last six months at the start rather than the full year. This hybrid approach (fix one end) also has that problem at the start, so you might as well simply revert to the naive. Naive start plus trend gives no added benefit.
But as I say, it is worse. Because the cold start (2008) gave a higher trend, and that then adds to the difference, even though it was not allowed to affect the starting point. They might argue that this doesn't apply if you add an a priori trend, which wouldn't be affected by 2008 cold. But still, that incorporates all the vagary of the choice of start value. And if they are talking about an a priori trend, then it makes no sense to form a constrained OLS. There are no degrees of freedom left.
Sou shows a table from the 2007 IPCC report in her latest post on the same subject. And yes, there are scenarios that have a change of around 3 C. But it is not the trend between 2008 and 2017, it's the change between 1980-1999 and 2090-2099 ( the centers of the periods are actually 105 years apart). The trend in the early 21st century is of course lower.
ReplyDeleteAnyway, we can skip OLS and the mysterious LAD regression and try difference between periods instead, eg 2013-2017 vs 2008-2012, which is 0.29 C vs 0.08 C. The 0.21 C difference suggests a century change of 4.2 C, so they will still lose by a mile..
I believe the goddess Miss Fortune is punishing their unfair comparison by giving them the absolutely warmest decadal trend in the UAH dataset..
UAH is probably the worst data set to use for a 10 year bet. The trend is all over the map depending on which decade you choose.
ReplyDeleteBut yeah. They definitely chose the wrong decade for this bet, regardless of the data set.
I believe their OLS estimate of 0.153 C/decade for UAH LT v6 *might* be a result of forcing the trend line to start at their 2007 average value (approx 0.16) at the beginning of 2008. When I do that I get an OLS trend of 0.156 C/decade, pretty close to theirs.
ReplyDeleteOf course it seems like by their reasoning they would want to force it to start at that value at 2007.5 not at 2008.0 since that's where they start the +0.3 C/decade model trend at. But if I do that, the OLS trend drops to 0.139 C/decade.
If that's what they're doing, I am guessing it's because they would say that for the sake of the forecast, the measured warming has to be relative to the warming at the time of the start of the forecast. Not a broken trend that jumps down 0.1 C below the starting temperature and then slowly rises back up.
Disclaimer: I have no idea what Armstrong & Green were actually thinking, or whether the close match between their reported OLS of 0.153 and mine (0.156) is purely coincidental.
"relative to the warming at the time of the start of the forecast"
Deleteshould be
"relative to the temperature at the time of the start of the forecast"
Ignoring the nonexistent "bet" and just seeking to answer the underlying question as honestly as possible ...
DeleteUse surface temperatures (GISTEMP), annual, and compare to the then-newly released CMIP3 A1B scenario temperatures. Apply a LOESS function with a variety of timescales (15, 20, 25, 30 years). Look at the temperature difference in the LOESS models between 2007 and 2017 (i.e., start vs end of decade).
For the CMIP3/IPCC A1B scenario, the increase is 0.20 to 0.22 over the decade depending on LOESS timescale (15 year to 30 year). Very close to the IPCC's statement about "0.2 C/decade" during the 2007-2027 period.
For GISTEMP, the increase is 0.29 to 0.21 over the decade (for the same 15 year to 30 year timescale LOESSes).
So the observed global mean surface temperature increase was comparable or more warm than in the model, even when measured in a smoothed time series to eliminate the ENSO cycle. This conclusion is conservative in that it ignores the issue of blended air/sea temperatures for model-obs comparison.
"I am guessing it's because they would say that for the sake of the forecast, the measured warming has to be relative to the temperature at the time of the start of the forecast"
DeleteYes, that seems likely. But it isn't consistent with OLS fitting. If you say that it has to be an a priori forecast, it should be an a priori trend, not a fitted one.
Of course, a real bettor on the other side would balk at piling an exaggerated trend (0.3/decade) on a warm year starting point. And while you say the "broken trend" dropped 0.1°C at the start, in fact that had happened by December 2007 (-0.04°C), almost 0.2°C below the 2007 mean. So it depends a lot on how you estimate the starting temp.
As I said in the post, if you are going to pin to a start estimate, you might as well just evaluate wrarming by end value (eg mean 2017) - start value.